
A t = P ( ( 1 + r n ) n t − 1 ) r n Substitute in the values. t = 18 Knowing P = 100, r = 0.05, n = 12 and t = 18, use the sum formula. r = 0.05 Identify n, the number of times the deposit will be made and the interest compounded each year. P = 100 Identify r, the annual interest rate, in decimal form. Identify P, the amount invested each month.

To find the Annuity formula, A t = P ( ( 1 + r n ) n t − 1 ) r n, A t = P ( ( 1 + r n ) n t − 1 ) r n, we need to identify P, r, n, and t. S = 5 9 We are asked to find the fraction form. S = 0.5 0.9 Multiply numerator and denominator by 10. a 1 = 0.5 Knowing a 1 = 0.5, r = 0.1, use the sum formula. 0.5555555555555 … Use place value to rewrite this as a sum. a n = a 1 r n − 1 We use the formula with a 1 = 3 and r = 2.

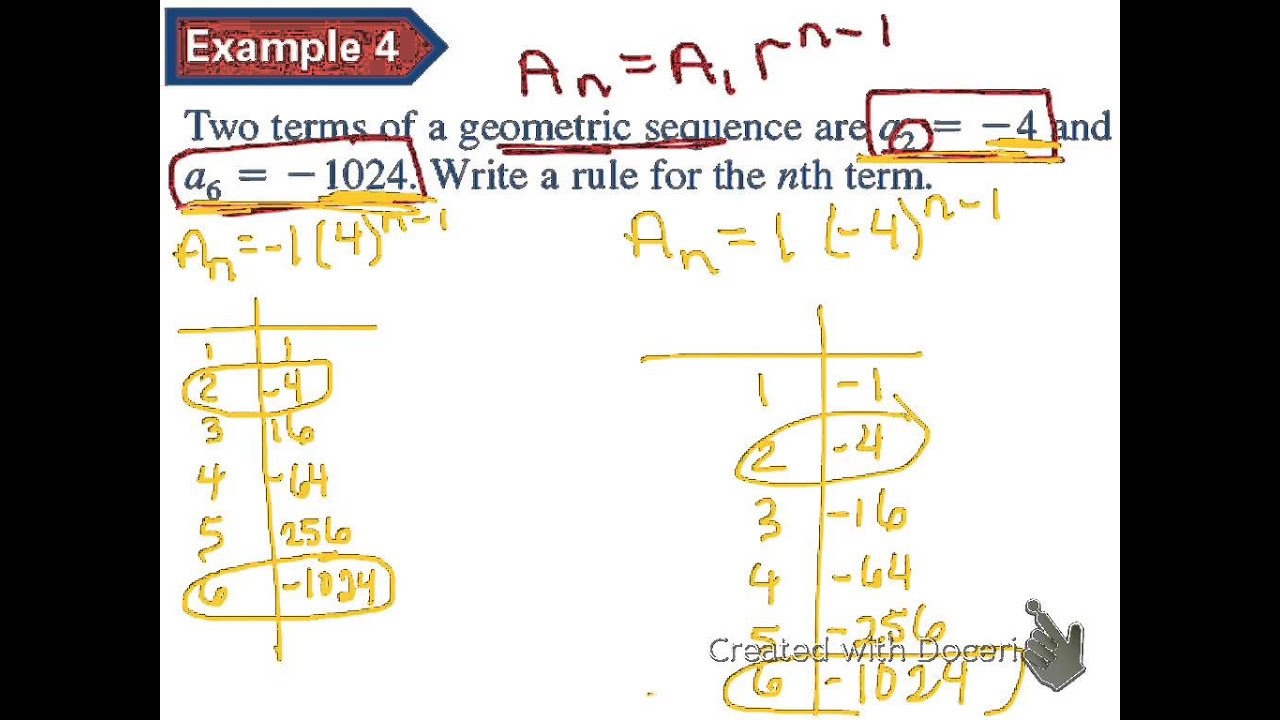
To find the twelfth term, we use the formula, a n = a 1 r n − 1, a n = a 1 r n − 1, and so we need to first determine a 1 a 1 and the common ratio r.ģ, 6, 12, 24, 48, 96, … The first term is three. In each term, the number of times a 1 a 1 is multiplied by r is one less than the number of the term. r or r 3 r 3) and in the fifth term, the a 1 a 1 is multiplied by r four times.In the fourth term, the a 1 a 1 is multiplied by r three times ( r In the third term, the a 1 a 1 is multiplied by r two times ( r In the second term, the a 1 a 1 is multiplied by r. The first term, a 1, a 1, is not multiplied by any r. We will then look for a pattern.Īs we look for a pattern in the five terms above, we see that each of the terms starts with a 1. Let’s write the first few terms of the sequence where the first term is a 1 a 1 and the common ratio is r.

Just as we found a formula for the general term of a sequence and an arithmetic sequence, we can also find a formula for the general term of a geometric sequence. Find the General Term ( nth Term) of a Geometric Sequence Write the first five terms of the sequence where the first term is 6 and the common ratio is r = −4. 27, 9, 3, 1, 1 3, 1 9, … Find the ratio of the consecutive terms. There is no common ratio.Ģ7, 9, 3, 1, 1 3, 1 9, … Find the ratio of the consecutive terms. To determine if the sequence is geometric, we find the ratio of the consecutive terms shown.įind the ratio of the consecutive terms.


 0 kommentar(er)
0 kommentar(er)
